|
Pure tone or pure note: sine curve
Consider the superposition of two harmonic waves at different
frequencies:
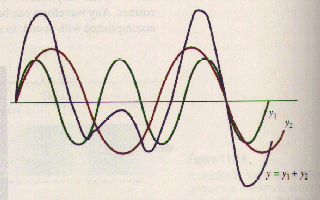
The resultant y = y1 + y2 (purple curve)
is periodic, but it is certainly not harmonic. Of course, to be harmonic
a disturbance must only have one frequency, and y does not.
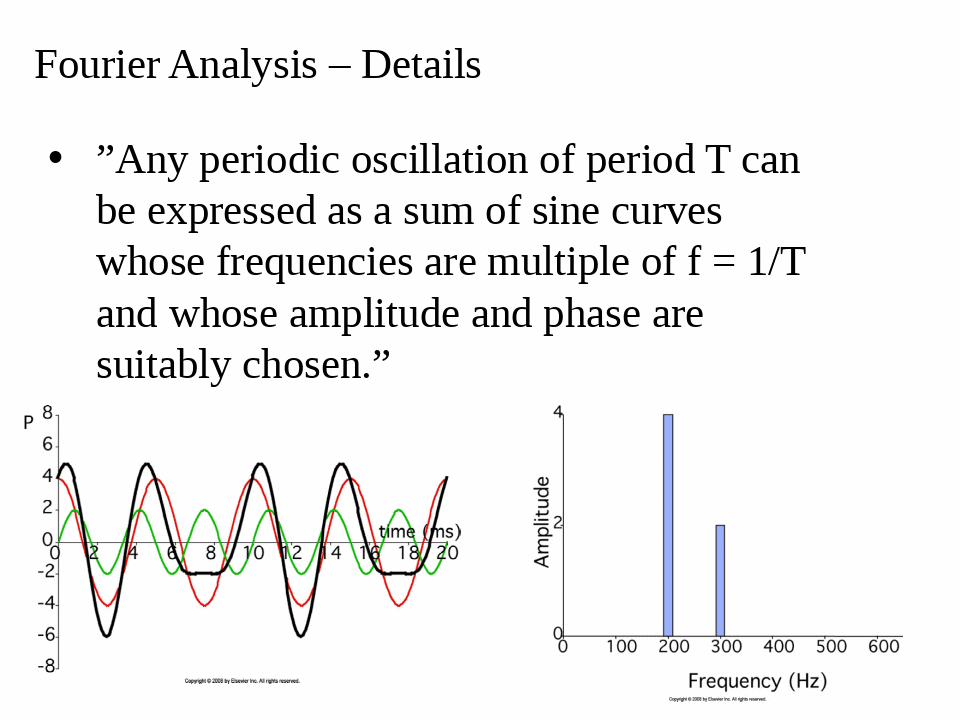
Superposition of several
sine waves
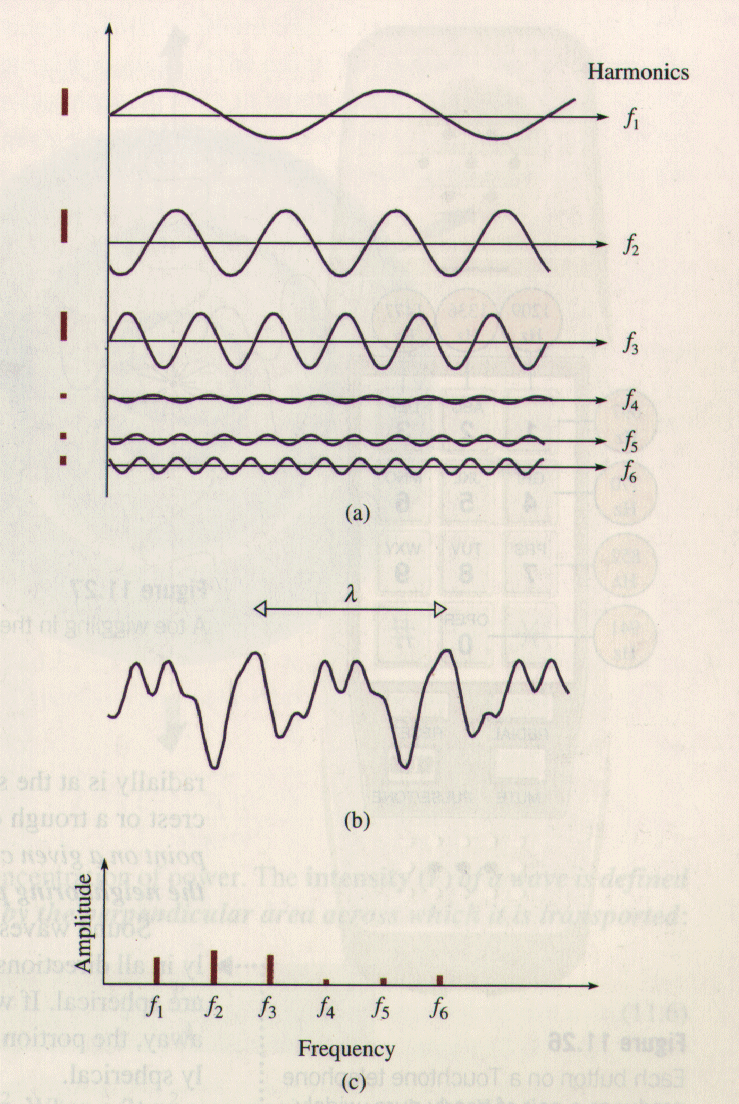
- (a) Six sinusoidal wave forms.
- (b) The result of combining these wave forms.
- (c) The associated spectrum. Each vertical line represents
the amplitude of the corresponding harmonic in (a). Note that
f2= 2 f1, f3=3 f1,
f4 = 4 f 1, and so forth.
Example:
A = 440 Hz
- 2. Harmonic: 880 Hz
- 3. Harmonic: 1320 Hz
- 4. Harmonic: 1760 Hz
Characteristics of different sounds
- Pure tone or pure note: sinus curve.
- Sound (music): superposition of several pure tones and
harmonics, complicated but regular pattern.
- Noise: irregular pattern
- Bang: spike
 Beats Beats
Superposition (sum) of 2 tones with slightly different frequencies
results in beats.
(The difference is less than about 10 Hz.)
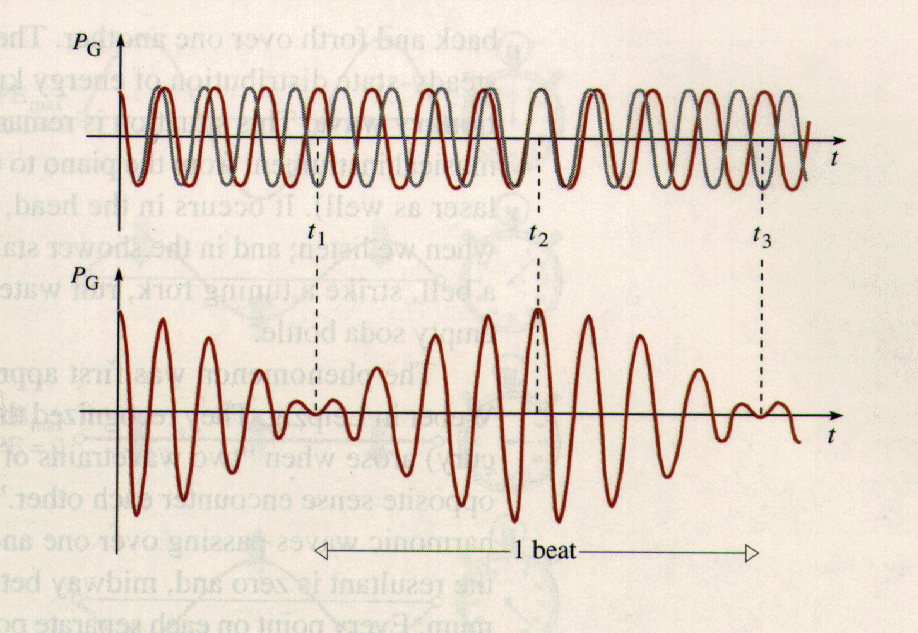
Note that at t1 and t3 the signals are
180o out-of-phase, while at t2 they are in-phase.
When two signals of different but nearly equal frequencies (f1
and f2) superimpose, they create a beat pattern, which is
true of sound as well as light. The intensity rises and falls with
a frequency equal to the difference (f1 - f2).
In equation form:
fbeat = | f1 - f2|
Here the absolute value, i.e. | f1 - f2|, means that either
f1 can be larger than f2 or vice versa. The beat frequency
does not distinguish which of the two frequencies is the larger one.
Here you can create
your own beats
The number of beats per second, or beat frequency, equals the difference
in frequency between the two sources.
 Sound Analysis - Mathematical Sound Analysis - Mathematical
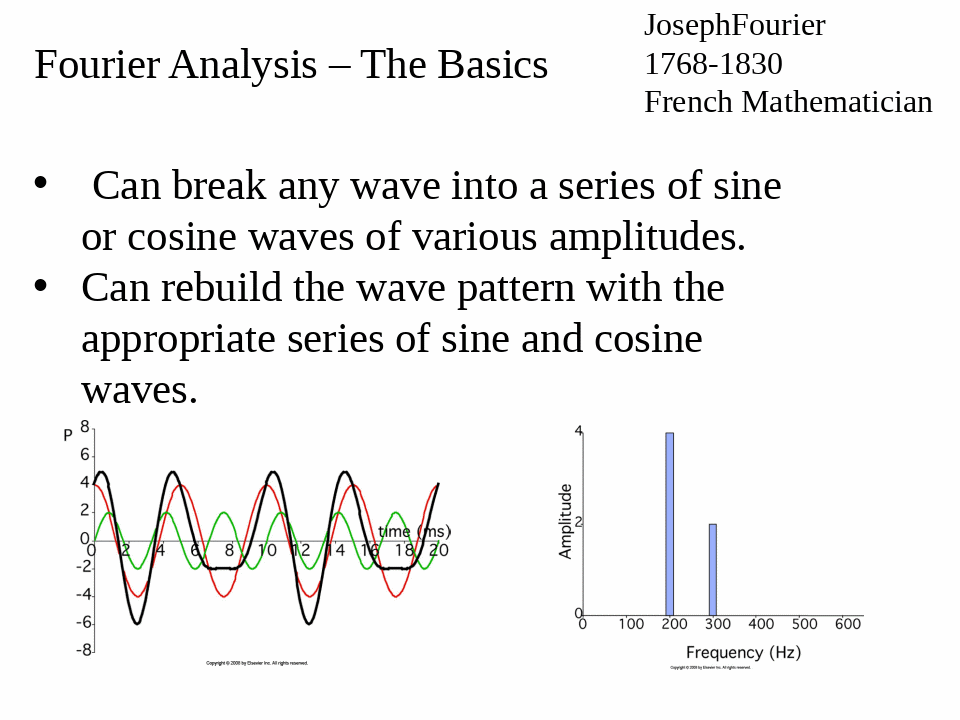




 Timbre Timbre
The auditory sensation that allows to distinguish between sounds is
timbre. Listening to a
flute, a trumpet, a saxophone, or a tuning fork, each producing the
same note (same pitch) at the same loudness, there is little difficulty
telling one from the other. The attribute that allows for this distinction
is the timbre, which depends primarily on the waveform - that is, the
frequencies present, their relative phases, and amplitudes. When the
same tones made by these various instuments are picked up by a
microphone and displayed on an oscilloscope, they are seen to be
substantially different. Similarly, the same note produced by
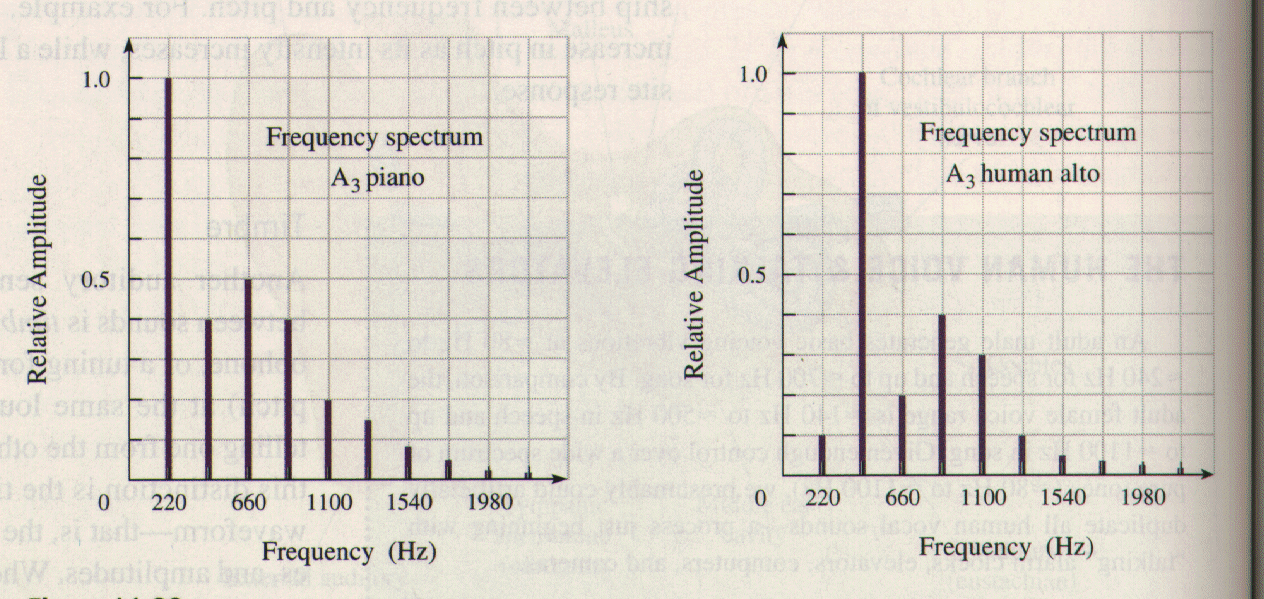
a piano and a singer have
distinctively different frequency spectra
and the ear immediately distinguishes the vocalist from the accompaniment,
even when the sounds are made at the same moment.
As example the frequency spectrum for the A3 (220 Hz)
note sounded by a piano and an
alto voice are shown here.
An actual tone will contain frequencies higher than the fundamental (or first
harmonic), and these are called overtones
. An overtone need not be a whole-numbered multiple of the
fundamental; that is, it need not be a harmonic.
Remember: Harmonics are exact multiples
of the fundamental.
 Overtones add richness
to the sound Overtones add richness
to the sound
It is the number of overtones
(whether they are harmonics or not) and
their relative amplitudes that more than anything else determines
timbre.
 Sound Quality Sound Quality
 Amplitudes of harmonics Amplitudes of harmonics
being added to the fundamentals
 Attack and decay of a note Attack and decay of a note
determine the characteristic of a tone
 Formant region of an
instrument Formant region of an
instrument
is the frequency region which is emphasized relative to other harmonics.
(This gives the unique sound to an instrument.)
 Vibrato
Vibrato
Periodic changes in the pitch of a musical tone.
 Tremelo
Tremelo
Periodic changes in the amplitude of a musical tone.
 Chorus effect Chorus effect
Many instruments play the same part in unison. -->
The sound quality is quite different from the individual instruments
due to superposition of many similar tones with slightly
different frequencies and tone qualities.
( sample)
 Audacity Audacity
Free - Open-source audio recording and modification progrom
download for mac
download for windows
download for linux
Import a wav file, e.g.
Effects: (Highlight region) e.g.
-- amplify
-- change pitch
-- equalizer
-- .....
Here are some samples to download:
-- Flute Solo
-- Cymbals
-- Keith Jarrett: The Köln Concert
Tutorial for Beginner:
Complete Tutorial Guide to Audacity for Beginners
Audacity 2.3.2 Manual
Audacity Tutorials

| 



 Sound
Sound
