Sinusoidal Volume Variations

In order to evaluate the performance of a Stirling engine, one must first determine the volume variations of the compression and expansion spaces with respect to the crankangle θ. Gustav Schmidt of the German Polytechnic Institute of Prague Published an analysis in 1871 in which he obtained closed form solutions of the Ideal Isothermal model for the special case of sinusoidal volume variations of the working spaces with respect to the cycle angle θ. In this section we wish to develop the sinusoidal volume relations Vc(θ) and Ve(θ) for Alpha, Beta and Gamma type engines.

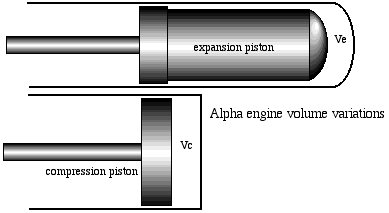
The Alpha type engine is the simplest of all three types in that there are two pistons, each independently controlling the respective working space. Thus the expansion piston only affects the volume variation of the expansion space Ve, and the compression piston only affects the volume variation of the compression space Vc. In the following figures we consider only the working spaces and not the interconnecting heat exchanger spaces.

The sinusoidal volume variations of the compression and expansion spaces are respectively as follows:

Vc = Vclc + Vswc (1 + cos θ) / 2

Ve = Vcle + Vswe (1 + cos(θ + α)) / 2

where Vcl and Vsw represent respectively clearence and swept volumes, and θ is the cycle angle. The constant angle α represents the phase advance of the expansion space volume variations with respect to the compression space volume variations.

Both Beta and Gamma type machines have more complex relations in that the movement of both the piston and the displacer affects the volume variation of the compression space Vc.

For the analysis that follows, we define the following variables:

Vc0 / Ve0 is the volume of the compression / expansion space (including clearence spaces) when both piston and displacer are in their mean positions.
Vca / Vea is the volume amplitude of the compression / expansion space. Thus Vswc = 2 Vea, Vswe = 2 Vea, Vclc = Vc0 - Vca, and Vcle = Ve0 - Vea.
Vpa / Vda is the volume amplitude in the compression space due to movement of the piston / displacer.
Ap / Ad is the cross sectional area of the piston / displacer in the compression space, i.e. the respective cylinder area minus the cross sectional area of the displacer rod.
Ae is the cross sectional area of the displacer in the expansion space.
xpa / xda is the amplitude of movement of the piston / displacer.

In the phasor diagram following

φ is the phase advance of the displacer with respect to the piston,
δ is the phase advance of the compression space volume with respect to the piston, and
α is the phase advance of the expansion space volume with respect to the compression space volume.

Vc = Vc0 - Vpa cos θ + Vda cos(θ + φ)

Expanding, we have

Vc = Vc0 + (Vda cos φ - Vpa) cos θ - (Vda sin φ)sin θ

Now consider the following phasor diagram

Vc = Vc0 + Vca cos(θ + δ)

Vc = Vc0 + Vca (cos θ cos δ - sin θ sin δ)

Equating like terms in the above equations for Vc, we obtain

Vca cos δ = Vda cos φ - Vpa

Vca sin δ = Vda sin φ

Thus finally

Vc = Vc0 + Vca cos(θ + δ) = Vclc + Vswc (1 + cos(θ + δ)) / 2

Ve = Ve0 + Vea cos(θ + δ + α) = Vcle + Vswe (1 + cos(θ + δ + α) / 2

where α = π + φ - δ

______________________________________________________________________________________

Stirling Cycle Machine Analysis by Israel Urieli is licensed under a Creative Commons Attribution-Noncommercial-Share Alike 3.0 United States License