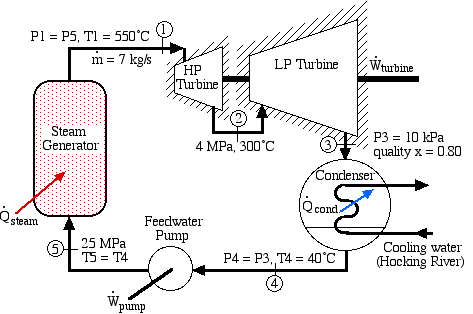
In an effort to decentralize the national power distribution grid, the following supercritical (25 MPa), coal fired steam power plant (modeled after the Gavin Power Plant in Cheshire, Ohio) has been proposed to service about 10,000 households in Athens, Ohio. It is to be placed close to the sewage plant on the east side of Athens and cooled by water from the Hocking river. We consider first a simplified system as shown below. Notice that we have replaced the "Boiler" with a "Steam Generator", since at supercritical pressures the concept of boiling water is undefined. Furthermore we have specifically split the turbine into a High Pressure (HP) turbine and a Low Pressure (LP) turbine since we will find that having a single turbine to expand from 25MPa to 10kPa is totally impractical. Thus for example the Gavin Power Plant has a turbine set consisting of 6 turbines - a High Pressure Turbine, an Intermediate Pressure (Reheat) turbine, and 4 large Low Pressure turbines operating in parallel.
Note that prior to doing any analysis we always first sketch the complete cycle on a P-h diagram based on the pressure, temperature, and quality data presented. This leads to the following diagram:
On examining the P-h diagram plot we notice that the system suffers from two major flaws:
The outlet pressure of the LP turbine at port (3) is 10 kPa, which is well below atmospheric pressure. In order to remove oxygen and other dissolved gases from the feedwater we need to introduce a de-aerator which requires the feedwater pressure to be above atmospheric pressure.
The quality of the steam at port (3) is 80%. This is unacceptable. The condensed water will cause erosion of the turbine blades, and we should always try to maintain a quality of above 90%. One example of the effects of this erosion can be seen on the blade tips of the final stage of the Gavin LP turbine. During 2000, all four LP turbines needed to be replaced because of the reduced performance resulting from this erosion. (Refer: Tour of the Gavin Power Plant - Feb. 2000)
The following revised system diagram corrects both flaws. The steam at the outlet of the HP turbine (port (2)) is reheated to 550 C before entering the LP turbine at port (3). Also the low pressure liquid condensate at port (5) is pumped to a pressure of 800 kPa and passed through a de-aerator prior to being pumped by the feedwater pump to the high pressure of 25 MPa.
This system is referred to as a Reheat cycle, and based on the data above is plotted on the P-h diagram as follows:
Thus we see that in spite of the complexity of the system, the P-h diagram plot enables an intuitive and qualitative initial understanding of the system. Using the methods described in Chapter 4b for analysis of each component, as well as the steam tables, determine the following:
a) Assuming that both turbines are adiabatic and neglecting kinetic energy effects determine the combined output power of both turbines [10.6 MW].
b) Assuming that both the condensate pump and the feedwater pump are adiabatic, determine the power required to drive the two pumps [-204 kW].
c) Determine the total heat transfer to the steam generator, including the reheat system [26.1 MW].
d) Determine the overall thermal efficiency of
this power plant. (Thermal efficiency (
th)
is defined as the net work done (turbines, pumps) divided by the
total heat supplied externally to the steam generator and reheat
system) [40 %].
e) Determine the heat rejected to the cooling water in the condenser [-15.7 MW].
f) Assume that all the heat rejected in the condenser is absorbed by cooling water from the Hocking River. To prevent thermal pollution the cooling water is not allowed to experience a temperature rise above 10°C. If the steam leaves the condenser as saturated liquid at 40°C, determine the required minimum volumetric flow rate of the cooling water [22.6 cubic meters/minute].
g) Discuss whether you think that the proposed system can be cooled by the Hocking river. You will need to do some research to determine the minimal seasonal flow in the river in order to validate your decision. (Hint- Google: Hocking River Flow)
______________________________________________________________________________________________
