Defining Entropy (S) through the Clausius Inequality

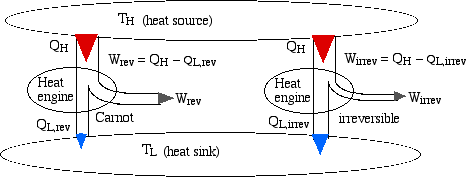
Consider two heat engines, one a reversible (Carnot) engine and the other an irreversible heat engine. For purposes of developing the Clausius Inequality we assume that both engines are sized to accept the same amount of heat Q_H from the thermal source. Thus since the irreversible engine must be less efficient than the Carnot engine, it must reject more heat Q_L,irrev to the thermal sink than that rejected by the Carnot engine Q_L,rev , as shown:

Consider first the reversible (Carnot) heat Engine. We saw in Chapter 5 that reversible heat transfer can only occur isothermally, thus the cyclic integral of the heat transfer divided by the temperature can be evaluated as follows:

Recall from Chapter 5 that whenever we considered the efficiency of a reversible heat engine, we went into "meditation mode", replacing the ratio of heat flows with the ratio of temperatures:

Notice from the above diagram showing the two heat engines that for an irreversible engine having the same value of heat transfer from the thermal source Q_H as the reversible engine, the heat transfer to the thermal sink Q_L,irrev> Q_L,rev.
Let Q_diff = (Q_L,irrev- Q_L,rev), then the cyclic integral for an irrevesible heat engine becomes:

Thus finally, for any reversible or irreversible heat engine we obtain the Clausius Inequality:

Defining the property Entropy - S

All properties (such as pressure P, volume V, etc) have a cyclic integral equal to zero.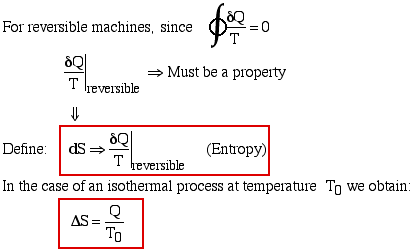

On to The Increase in Entropy Principle

______________________________________________________________________________________

Engineering Thermodynamics by Israel Urieli is licensed under a Creative Commons Attribution-Noncommercial-Share Alike 3.0 United States License