
Water rockets have been fascinating children and adults alike throughout the world for some time now, possibly because they are conceptually simple, requiring an ingenious combination of "exotic" components such as plastic soda pop bottles, water, a bicycle pump - and their behavior is totally unpredictable.
We were directed by Dr Johannes Knapp of DESY Southern Germany to a remarkable youtube video of the first documented manned water rocket launch in Japan which made us realize the great potential of the water rocket as the possible transportation system of the future.
During 2004 the late Dr Ken Halliday decided to use Water Rockets as the theme project for the ME100 freshman class "Introduction to Mechanical Engineering" - assisted by Dr Andy Foley and the ME398 Junior Lab. Dr. Halliday took an amazing split-second photographic sequence showing Dr Foley demonstrating a typical launch sequence of one of the student rockets.
We searched the web for a suitable analysis to use and decided to adopt the approach presented by Dr. Peter Nielsen of the University of Queensland in Australia. Dr Nielsen has presented a summary of the equations as well as the results of a simulation (Nielsen_Rocket.pdf [164k file]), which we will use as the default values in our exercises. In the following we have developed the rather complex analysis leading to the differential equation for the compressed air volume variation, essentially following the presentation by Dr. Nielsen. In subsequent analyses we continue to develop the differential equations for upwards acceleration, velocity and height attained as well as the numerical integration technique for evaluating these variables as functions of time.
Consider a typical water rocket such as the
"Rolling Rock" Rocket, proudly demonstrated by students
of a previous ME100 class. (rumour has it that beer was used instead
of water in this rocket).

Based on the Water Rockets page of Dr Knapp, the cross section of a typical water rocket illustrating the principle of operation is shown in the following diagram:
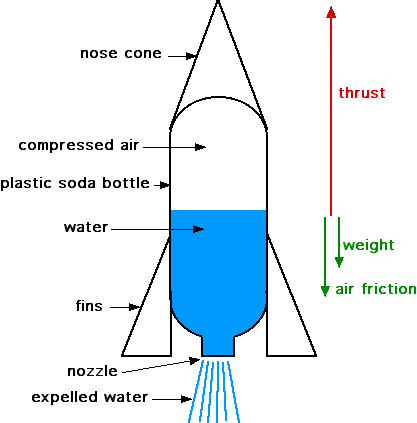
Thus the compressed air in the bottle forces the water through a nozzle (bottle neck) which produces the thrust required to accelerate the bottle (hopefully) vertically upwards. We determine the time derivative of its vertical velocity by Newton's second law of motion:
where:
m is the instantaneous total mass of the rocket [kg]
u is the upwards velocity [m/s]
Fthrust is the thrust forsce (due to the expelled water) [N]
Fdrag is the drag force from the surrounding air [N]
g is the acceleration due to gravity [9.81 m/s2]
The thrust force is proportional to the exhaust mass flow through the nozzle times the velocity of the exhaust relative to the rocket.
where:
is the rate of mass flow of the expelled water [kg/s]
uex is the exhaust velocity of the expelled water through the nozzle [m/s]
is the density of water [1000 kg/m3]
AN is the area of the nozzle [m2]
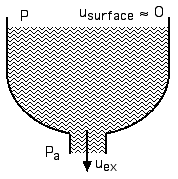 |
Bernoulli's equation can be derived from the energy equation applied to the water flowing through the nozzle. It relates the kinetic energy of the exhaust water to the compressed air pressure applied at the water surface. Neglecting potential energy terms, we have: |
Combining equations (2) and (3) above we obtain:
We now continue with Page 2 of the water rocket analysis, leading to the compressed air volume variation differential equation. Solving this equation will allow us to evaluate the rocket performance, leading ultimately to the altitude attained by the rocket.
______________________________________________________________________________________

Engineering Thermodynamics by Israel Urieli is licensed under a
Creative Commons Attribution-Noncommercial-Share
Alike 3.0 United States License