
In this chapter various relevant concepts and definitions are introduced, and will be used throughout the course.
Thermodynamics is the science of energy, including energy storage and energy in transit. The Conservation of Energy Principle states that energy cannot be created or destroyed, but can only change its form. The three forms of energy storage of greatest interest to us are Potential Energy (PE), Kinetic Energy (KE), and Internal Energy (U), which we introduce below. The two forms of energy in transit that we consider are Work (W) and Heat (Q), and the interactions between these various forms of energy are defined in terms of the First Law of Thermodynamics, which we introduce in Chapter 3.
In this course we use the International System (SI) units exclusively, with occasional lapses. In the US this is an ongoing battle causing much confusion in the global technical environment (refer to wikipedia on this subject). Even the National Council of Examiners for Engineering and Surveying (NCEES) seems to be confused at this point - the Fundamentals of Engineering (FE) Reference Handbook and exam contain exclusively SI units and then, when you reach maturity and are ready to take the Professional Engineering (PE) exam, you find that the English system of units (USCS) is acceptable, and in some cases used exclusively. This confusion reached a new climax when in the Fall of 1999, NASA's $125 million Mars Climate Orbiter broke up in the Martian atmosphere, because scientists in one of NASA's subcontractors failed to convert critical data from the English sytem to the SI system of units.
We begin with Newton's Second Law, as follows:
|
The Weight of a body is the force acting on that body due to the acceleration due to gravity (g = 9.807 [m/s2]), in accordance with the Universal Theory of Gravitation developed by Isaac Newton. Legend has it that Newton was inspired by an apple falling on his head, as is shown in the delightful animation by Mike Guidry of the University of Tennessee. Well, this legend is extremely relevant, since the
weight of a small apple is approximately one Newton. Furthermore,
the mass of a plastic bottle containing one liter of water is
approximately one kilogram. |
|
At this point we note that there is a confusion about mass and weight units, in particular with the English system of units. This came about because of the decision to define mass and weight independently as 1 lb (pound), when in fact they are related through Newton's Second Law. In order to justify this one has to separately define a pound mass (lbm) and a pound force (lbf), thus since the acceleration due to gravity g = 32.2 [ft/s2] we have:
One attempt to solve this paradox has been the introduction of a new unit of mass, the slug, thus:
1 slug = 32.2 lbm
however I challenge anyone to go to the grocery store and request a slug of potatos.
We now consider the work done (W), the energy in transit requiring both the applied force (F) and movement (x). If the force (F) is constant over the distance moved (x) then the work done is given by:
However, in general the force (F) is not constant over the distance x, thus we need to sum all the incremental work processes taking into consideration the variation of the force (F). This leads to the equivalent integral form for determining work done (W) as follows:
Over the years we have developed a basic Units Survival Kit (for the SI challenged) in order to help convert between the USCS (English) system and the SI (International) system of units, as well as to develop a feel for the magnitudes of the various units.
Quick Quiz - we all know
(from reading our speedometers) that 50 mph is equivalent to 80
km/hr.
1. What is the accuracy of this conversion?
2. Use this
information to show that 9 mph is equivalent to 4 m/s.
We find that with the above survival kit we can determine many unit conversions between SI & English units, typically as demonstrated in the following block:
As we progress and learn new concepts we will add to this Survival Kit.
We introduce the various forms of energy of interest to us in terms of a solid body having a mass m [kg]. These include potential, kinetic and internal energy. Potential energy (PE) is associated with the elevation of the body, and can be evaluated in terms of the work done to lift the body from one datum level to another under a constant acceleration due to gravity g [m/s2], as follows:
Kinetic energy (KE) of a body is associated with its
velocity
![]() [m/s]
and can be evaluated in terms of the work required to change the
velocity of the body, as follows:
[m/s]
and can be evaluated in terms of the work required to change the
velocity of the body, as follows:
Internal energy (U) of a body is that associated with the molecular activity of the body as indicated by its temperature T [°C], and can be evaluated in terms of the heat required to change the temperature of the body having a specific heat capacity C [J/kg.°C], as follows:
In order to gain an intuitive appreciation for the relative magnitudes of the different forms of energy we consider the (tongue-in-cheek) example of an attempt to cook a turkey by potential energy. The turkey is brought to the top of a 100 m building (about 30 stories) and then dropped from the ledge. The potential energy is thus converted into kinetic energy, and finally on impact the kinetic energy is converted into internal energy. The increase in internal energy is represented by an increase in temperature, and hopefully, if this experiment is repeated enough times the temperature increase will allow the turkey to cook. This remarkable experiment was first reported in 1987 by R.C.Gimmi and Gloria J Browne - "Cooking with Potential Energy" .
What a disappointment! At 0.33°C per fall it will require repeating the experiment 600 times just to reach the cooking temperature of 200°C.
For purposes of analysis we consider two types of Thermodynamic Systems:
Closed System
- usually referred to as a System
or a Control
Mass. This type of system is
separated from its surroundings by a physical boundary. Energy in
transit in the form of Work or Heat can flow across the system
boundary, however there can be no mass flow across the boundary. One
typical example of a system is a piston / cylinder device in which
the system is defined as the fixed mass of fluid contained within
the cylinder.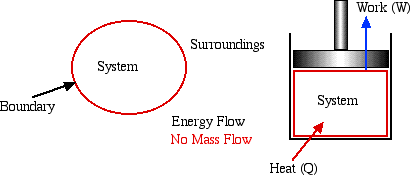
Open System - usually
referred to as a Control
Volume. In this case, in addition to
work or heat, we have mass flow of the working fluid across the
system boundaries through inlet and outlet ports. In this course we
will be exclusively concerned with steady flow control volumes, in
that the net mass of working fluid within the system boundaries
remains constant (ie mass flow in [kg/s] = mass flow out [kg/s]).
The following sections refer mainly to systems - we will consider
control volumes in more detail starting with Chapter
4a.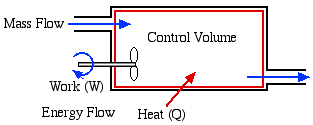
The closed system shown above can be defined by its various Properties, such as its pressure (P), temperature (T), volume (V) and mass (m). We will introduce and define the various properties of thermodynamic interest as needed in context. Furthermore the properties can be either Extensive or Intensive (or Specific). An extensive property is one whose value depends on the mass of the system, as opposed to an intensive property (such as pressure or temperature) which is independent of the system mass. A specific property is an intensive property which has been obtained by dividing the extensive property by the mass of the system. Two examples follow - notice that specific properties will always have kilograms (kg) in the units denominater.
One often used exception to the above definitions is the concept of Specific Weight, defined as the weight per unit volume. We will not be using this concept throughout this text.
The State of a system is defined by the values of the various intensive properties of the system. The State Postulate states that if two independent intensive property values are defined, then all the other intensive property values (and thus the state of the system) are also defined. This can significantly simplify the graphical representation of a system, since only two-dimensional plots are required. Note that pressure and temperature are not necessarily independent properties, thus a boiling liquid will change its state from liquid to vapor at a constant temperature and pressure.
We assume that throughout the system Equilibrium conditions prevail, thus there are no temperature or pressure gradients or transient effects. At any instant the entire system is under chemical and phase equilibrium.
A Process is a change of state of a system from an initial to a final state due to an energy interaction (work or heat) with its surroundings. For example in the following diagram the system has undergone a compression process in the piston-cylinder device.
The Process Path defines the type of process undergone. Typical process paths are:
Isothermal (constant temperature process)
Isochoric or Isometric (constant volume process)
Isobaric (constant pressure process)
Adiabatic (no heat flow to or from the system during the process)
We assume that all processes are Quasi-Static in that equilibrium is attained after each incremental step of the process.
A system undergoes a Cycle when it goes through a sequence of processes that leads the system back to its original state.
The basic unit of pressure is the Pascal [Pa], however practical units are kiloPascal [kPa], bar [100 kPa] or atm (atmosphere) [101.32 kPa]. The Gage (or Vacuum) pressure is related to the Absolute pressure as shown in the diagram below:
The basic method of measuring pressure is by means of a Manometer, as shown below:
The atmospheric pressure is measured by means of a Mercury Barometer as follows:
Solved Problem 1.1 - Using a Barometer to determine the Height of a Building This solved problem allows us to determine the height of a building in terms of the difference in atmospheric pressure between the top and bottom of the building. Note that according to legend, when this problem was put to Nobel Prize Winner Niels Bohr (while still a student), he came up with an interesting response, as shown in the Australian website of the Virtual Teacher (this page no longer available).
Temperature is a measure of molecular activity, and a temperature difference between two bodies in contact (for example the immediate surroundings and the system) is the driving force leading to heat transfer between them.
Both the Fahrenheit and the Celsius scales are in common usage in the US, hence it is important to be able to convert between them. Furthermore we will find that in some cases we require the Absolute (Rankine and Kelvin) temperature scales (for example when using the Ideal Gas Equation of State), thus we find it convenient to plot all four scales as follows:
Notice from the plot that -40°C equals -40°F, leading to convenient formulas for converting between the two scales as follows:
Quick Quiz - The temperature in Chicago in winter can be as low as 14°F. What is the temperature in °C, K, and °R. [-10°C, 263 K, 474°R] Note that by convention 263 K is read "263 Kelvin," and not "263 degrees Kelvin".
______________________________________________________________________________________
![]()
Engineering Thermodynamics by Israel
Urieli is licensed under a Creative
Commons Attribution-Noncommercial-Share Alike 3.0 United States
License