Thanks to Kris Dambrink from Imtech.nl (currently inactive), for making me aware of this alternative approach to adapting an Open Feedwater Heater to a steam power plant (4 Feb 2010)
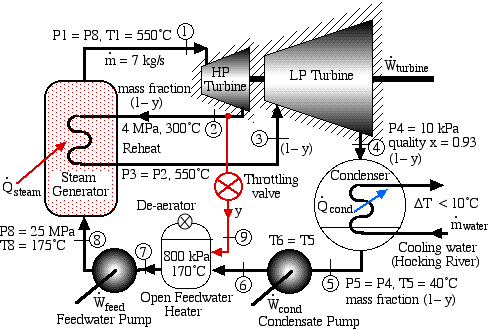
This Solved Problem is an alternative extension of Solved Problem 4.1 in which we extend the de-aerator by tapping steam from the outlet of the High Pressure turbine and reduce the pressure to 800 kPa by means of a Throttling Control Valve before feeding it into the de-aerator. This allows one to conveniently convert the de-aerator into an Open Feedwater Heater without requiring a bleed tap from the Low Pressure turbine at exactly the de-arator pressure, as shown in the following diagram:
Note that prior to doing any analysis we always first sketch the complete cycle on a P-h diagram based on the pressure, temperature, and quality data presented on the system diagram. This leads to the following diagram:
On examining the P-h diagram plot we notice the following:
A mass fraction of the steam y is tapped from the outlet of the HP turbine (2) and passed through the throttle such that its pressure is reduced to that of the deaerator (9). It is then mixed with a mass fraction (1-y) of the liquid water at station (6). The mass fraction y is chosen to enable the fluid to reach a saturated liquid state at station (7).
The feedwater pump then pumps the liquid to station (8), thus saving a significant amount of heat from the steam generator in heating the fluid from station (8) to the turbine inlet at station (1). It is true that with a mass fraction of (1-y) there is less power output due to a reduced mass flow rate in the LP turbine, however the net result is normally an increase in thermal efficiency.
Thus once more we see that in spite of the complexity of the system, the P-h diagram plot enables an intuitive and qualitative initial understanding of the system. Using the methods described in Chapter 4b for analysis of each component, as well as the steam tables for evaluating the enthalpy at the various stations (shown in red), and neglecting kinetic and potential energy effects, determine the following:
a) Assuming that the open feedwater heater is
adiabatic, determine the mass fraction of steam y required to be
bled off the outlet of the HP turbine which will bring the fluid
from station (6) to a saturated liquid state in the deaerator. [y
= 0.20]
We first need to evaluate the
enthalpy of the fluid at station (9) after passing through the
throttling control valve:
Thus we find that for an ideal throttle the enthalpy h9 = h2 independent of the pressure drop, allowing us to conveniently draw the throttling process as a vertical line on the P-h diagram. We now determine the mass fraction y by considering the mixing process in the open feedwater heater as follows:
Notice that we can estimate this value of y directly from the P-h diagram by simply measuring the enthalpy differences (h7 - h6) and (h9 - h6) with a ruler.
b) Assuming that both the condensate pump and
the feedwater pump are adiabatic, determine the power required to
drive the two pumps [235
kW].
On examining the system diagram
above we noticed something very strange about the feedwater pump.
Until now we considered liquid water to be incompressible, thus
pumping it to a higher pressure did not result in an increase of its
temperature. However on a recent visit to the Gavin Power Plant we
discovered that at 25MPa pressure and more than 100°C water is no
longer incompressible, and compression will always result in a
temperature increase. We cannot use the simple incompressible liquid
formula to determine pump work, however need to evaluate the
difference in enthalpy from the Compressed
Liquid Water tables, leading to the
following results:
c) Assuming that both turbines are adiabatic, determine the new (reduced) combined power output of both turbines. Recall from Solved Problem 4.1 that the power output of the turbines was found to be 10.6 MW if no steam is bled from the LP turbine [8.98 MW]
Thus as expected we find that the net power output is slightly less than the previous system without the turbine tap. However power control is normally done by changing the feedwater pump speed, and we normally find a liquid water storage tank associated with the de-aerator in order to accomodate the changes in the water mass flow rate. In our case we simply need to increase the water mass flow rate from 7 kg/s to 8.25 kg/s in order to regain our original power output.
d) Determine the total heat transfer to the steam generator, including the reheat system [21.4 MW].
e) Determine the overall thermal efficiency of this power plant. (Thermal efficiency (ηth) is defined as the net work done (turbines, pumps) divided by the total heat supplied externally to the steam generator and reheat system) [41 %].
f) Determine the heat rejected to the cooling water in the condenser [-12.6 MW].
g) Assume that all the heat rejected in the condenser is absorbed by cooling water from the Hocking River. To prevent thermal pollution the cooling water is not allowed to experience a temperature rise above 10°C. If the steam leaves the condenser as saturated liquid at 40°C, determine the required minimum volumetric flow rate of the cooling water [18.1 cubic meters/minute].
Note that it is always a good idea to validate ones calculations by evaluating the thermal efficiency using only the heat supplied to the steam generator and that rejected by the condenser.
Discussion: Thus we find
that the open feedwater heater did in fact raise the efficiency from
40% to 41%. This may not seem like a significant amount, however all
the basic components were already in place, since without a
de-aerator
the dissolved oxygen and carbon dioxide in the feedwater
will cause serious corrosion damage in the boiler. Furthermore, if
the reduction in power output is not acceptable, then it can be
easily remedied by increasing the mass flow rate in the system
design. Note that this is a contrived example in order to demonstrate
that no matter how complex the system is, we can easily plot the
entire system on a P-h diagram
and obtain an immediate intuitive understanding and evaluation of the
system performance. It is helpful to check each value of enthalpy
read or evaluated from the steam tables and compare them to the
values on the enthalpy axis of the P-h
diagram.
___________________________________________________________________________________________