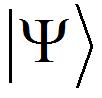Physics 611   Winter Quarter 2010              Instructor: Justin Frantz
Ohio University
T.A. Bing Xia


Graduate Quantum Mechanics


Syllabus    (in MS Word here)


Assignments
Problem Set #1:    Due 1/15/2010    In class, or by 4pm at colloquium.
 Sakurai Problems:      1) 1.2     2)  1.4: a. & b. (not c or d)   3) 1.12
 Essay Question:   [ based on Required Reading 1) ]:  Write a single paragraph of at least 6 sentences explaining your answers the following set of questions:
What is not generally agreed upon about the "Shrodinger's Cat" thought experiment?   Do you believe that the cat exists in both states simultaneously? (explain what two states I mean, if you haven't answered this already)   In our Stern-Gerlach "thought experiment"  at which of the following points does the wave function collapse of the Ag atom occur ? (Justify your answer)  1) Before the magnetic (B) field?  2) While passing through the B field?  3) After passing through the B field, but before it hits the screen.  4)  When it hits the screen.   5) Some point after it hits the screen.   Relate your choice (or one of the other alternatives, or the experiment as a whole) to Shrodinger's Cat experiment--meaning for at least that point, identify the analogous point in the Shrodingers Cat experiment.   Finally, how does the situation change when we remove the screen?

Problem Set #2:    Due Friday 1/22/2010  In class, or by 4pm at colloquium or in my mailbox. 
             Sakurai Problems:   1) 1.5     2) 1.11      3) 1.17    4)  1.18 a.  

Problem Set #3:    Due Friday 1/29/2010  In class, or by 4pm at colloquium or in my mailbox. 
             Sakurai Problems:   1) 1.19   2) 1.23 c   3) 1.24    4)  1.26
             
Problem Set #4:    Due Monday 2/8/2010  In class, or by 5pm in Edwards Accelerator Lab mailbox.
            Do required reading see below (potentially at least most is due before 2/8)
            Sakurai Problems:   1) 1.21 but only do it for the ground state  2) 1.28 b. & c.  3) 1.29 a.  4) 1.33 (hint: answer/understand b) first, considering what the operator should do on a p basis ket |p>  (what's p(x|p>)? ),  then forming the infinitesimal version of b) prove a.)(i) as we did in class for <x|p|\alpha>. 

Problem Set #5:  Due Friday 2/12/2010  at the usual time/locations.
            1)  Parts a. & b. of the problem (2) in the following document (from C. Elster's 2005 Phys 611 class)
            2)  Read Sakurai section 2.3 (only until "Time Development of the Oscillator" pp. 89-94)
a)  Give a bra/ket expression of the operator N using outer products of its eigenkets |n> (hint: it has an infinite number of terms). 
b)  Give a similar bra/ket expression (using outer products of eigenkets |n>) of the annihilation    operator a  (hint:  think of the explicit general bra/ket form of the transformation operator U.) in which a does not explicitly appear.

Problem Set #6:    Due Wed. 2/24/2010  at the usual time/locations.
             Sakurai Problems:   1) 3.9   2) 2.1   3) 2.3
             Essay Question:  Each question: 3 sentences OR LESS!  (i) Explain why the 50/50 mixture of 2 pure polarized Sz +/- samples becomes a completely unpolarized mixture.  (ii) Is there (or should there be) a physical difference between a 50/50 mixture of Sz +/- and a 50/50 mixture of Sy+/-?  (iii) Do you think the "mixed ensemble" interpretation or the "mixed state (vs wave function) of a single quantum system" interpretation works better for describing this property of spin systems?


Problem Set #7:    Due Fri. 3/5/2010  at the usual time/locations.
             Sakurai Problems:   1) 2.11  2) 2.22  
               3) Imagine the same problem as 3) (Sak 2.22)  for all time until t=0 (ie for t < 0).  However at t=0, the coefficient of the delta function changes to v0'.  What is the probability that the bound particle will stay bound in the new potential?  To evaluate this make the "sudden approximation" which is simply that the probability will be given by the projection (norm squared) of the old state onto the new state.  
Problem 4 & 5 now due Tues 3/9, usual time/locations.
               4) Use the WKB approximation to estimate the energy levels for 1-D particle in the box (same as Sakurai problem 1.21).    Show that resulting approximate energy levels can be written EWKB = g(n)En, where En is the exact result, and g(n) is some fairly simple function of n.  Explain in what limit of n is the WKB result accurate and why this makes sense given the basic ideas of the WKB approximation.
               5)  Do the following WKB scattering problem.

Problem Set #8:    Due by Sat. 3/13/2010  at the review session.
             Sakurai Problems:   1) 2.36 
             Liboff Problems:      2) 14.1    3)  14.6
                 


Links
Required Reading

1)    Read Sakurai 1.2, 1.3, and the first 2 pages of 1.4 first, then read: Wikipedia: Measurement in Quantum Mechanics;  Don't worry about understanding fully everything.  Especially, just skim, or skip over the "Quantitative Details" section (as this is what we will be learning/studying in the next ~month).  Under "Wave Function Collapse"  completely skip the "Measurements of the second kind" & "Decoherence in quantum measurement" subsections, but you should be able to understand the rest of that section.  In general, don't worry about following other links interspersed in the text if you don't know what something is and there's another Wikipedia page explaining it.  I certainly don't expect you to understand this page to the point that you know what every word on the page.  But following a few of them down "one level" or so will probably help you understand what is there.    Finally please also read Wikipedia: Shrodinger's Cat.

2)  By 2/5/2010 : (In addition to all of Chapter 1) In this order: Sakurai section 3.9 and then 3.4 up to p. 181 just before section "Time Evolution of Ensembles" (not including that).

3)  By 2/17/2010 : Sakurai  Chapt 2, Sections 2.1-2.2

4)  By 3/1/2010 : Sakurai  Chapt 2, Sections 2.4  Liboff 7.5, 7.6;   by ~3/2: 2.5-6  Liboff 7.5, 7.6, 7.10, 7.11:  the rest of Liboff Ch 7 should be considered useful except for the sections regarding the SHO.

5)  By 3/10/2010 :  Liboff 14.1, 14.2 & 14.4  Sakurai Ch 7 may be a good optional reference for certain of these topics. 

Optional Reading

1)    Google "Index notation":  find a good reference that includes matrices.   Good ones I found:
http://www.physicsforums.com/showthread.php?t=198712 cached here.
http://web.mit.edu/8.07/www/IndexNotation.pdf cached here: here.
2)  Explanation of Generating Functions in Classical Mechanics: Wikipedia:Canonical Transformations.  OK explanantion, but in the end Sakurai's mention of this on page 46 turns out to be not very helpful at all--thus I provide this reference only to prove that to yourself, if you care to.  "Generating Functions" in classical mechanics don't bear any obvious resemblence to "generators" in group theory, and it will not be fruitful in other cases to follow this fairly contrived scheme of "motivating" quantum mechanical expressions.
3) Quantiki Wiki : GREAT REFERENCE to all things Quantum:  especially Product Spaces/States, Entanglement, Mixed States, etc...
3)  Wikipedia:  Airy Functions (the special functions used to do the matching in the WKB approx)
4) A great explanation of why the Lagrangian and Hamiltonian take the forms given in Sakurai in the case of electrodynamic fields e.g. vector potentials A.  It also shows that  the "p" in  the Hamiltonian definition, is actually the generalized momentum mv + eA/c, not just mv.  See page 27.   You can also see latter point explained without reference to special relativity on the next Wikipedia link too, which explains Hamiltonian Mechanics--this part is way at the bottom (search for "Charged particle in an electromagnetic field").
5) Wikipedia:  Hamiltonian Mechanics explains the basics (a great refresher!) of both Hamiltonian and Lagrangian formulations of classical mechanics
 

Practice Problems:
    The following few problems can be evaluated as good practice for midterm.  Solutions.




Class Notes
All Notes Compiled into One File (first half) (~pre mid-term)
Notes for Unit V: "Unfinished Business with Formalism"
All Notes Compiled into One File (second half)  (~post mid-term starting with the SHO)  


Prakash Derivation of Hamilton Jacobi Equation in Quantum Wave Mechanics